In mechanics, the normal force Fn is the component, perpendicular to the surface (surface being a plane) of contact, of the contact force exerted on an object by, for example, the surface of a floor or wall, preventing the object to fall. For example, consider a person standing still on the ground, in which case the ground reaction force reduces to the normal force. In another common situation, if an object hits a surface with some speed, and the surface can withstand it, the normal force provides for a rapid deceleration, which will depend on the flexibility of the surface.
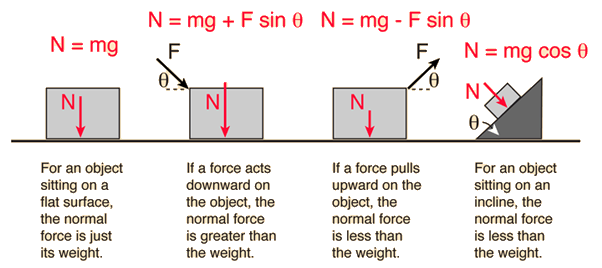
In a simple case such as an object resting upon a table, the normal force on the object is equal but in opposite direction to the gravitational force applied on the object (or the weight of the object), that is, N = mg, where m is mass, and g is the gravitational field strength (about 9.81 m/s2 on Earth). The normal force here represents the force applied by the table against the object that prevents it from sinking through the table, and requires that the table is sturdy enough to deliver this normal force without breaking.
Where an object rests on an incline, the normal force is perpendicular to the plane the object rests on. Still, the normal force will be as large as necessary to prevent sinking through the surface, presuming the surface is sturdy enough. The strength of the force can be calculated as:

where N is the normal force, m is the mass of the object, g is the gravitational field strength, and θ is the angle of the inclined surface measured from the horizontal.
The normal force is one of several forces which act on the object. In the simple situations so far considered, the most important other forces acting on it are friction and the force of gravity.